A maths question posed to 16 year olds in England caused a
Twitterstorm, as teenage wrath came down upon the examiners for being deliberately
obscure, oblique and irrelevant. A petition has reached 15,000 signatures (impressive number),
asking for the grade boundaries to be changed. Something freaked these students and it's worth reflecting on what could have gone wrong. After all, in previous exams, impossible to answer questions have been set.
The maths Taliban would love every child to be able, like them, to
solve this conundrum and their reaction has been to blame the students and claim that here's nothing wrong here. But i think there is fire behind this smokescreen - it is a poor test item. It is also a
touchstone for educational debate.
1. Bait and switch
Although it sets you up with a probability question, they
bait you with probability, then switch to algebra and throw in a quadratic. To understand why this is a
problem you have to understand that the maths GCSE curriculum is taught in
silos – number, algebra, ratios, geometry, probability and statistics. Maths is not (or
rarely) taught in terms of the broader mathematical thinking skills required to answer this type of question. Kids are taught to identify the ‘type’ of question in relation to the
curriculum categories they’ve been taught and apply the rules they’ve been
taught in that one domain. I understand that this is not right but it's the reality. That, in iteself, I suspect, explains the uproar.
2. Final probabilty is given to problem
Rather puzzlingly, they use the all too
predictably infantile, Beano-like, how many sweets are in the bag (really - sweets?) story. This is a bit pathetic and an artefact that seems to only exist in the minds of exam setters. They are then
confused by being given a known probability (1/3). This sets up dissonance in the
mind of the learner. They think - hold on – I though we were being asked to find out how
many sweets are in the bag (solve a probability problem) now I’m being given a
final probability – that doesn’t make sense. By giving the final probability in the
question, you confuse the student. They’re asked to fill out the middle of a
sandwich, which is rarely real, and not what they’ve been doing for the last
four years. In fact it’s rare in mathematics. I suspect that a lot of students would have been panicked by this on first reading.
3. Trap
As an assessment item it is poor, as it lays a trap for
students. The appearance of a quadratic equation in the question n2 – n – 90 = 0 suggests that this needs to be solved - that's almost what asked for in the final statement.
Most of the young people sitting this exam would have been drilled in solving
equations like this. Again, I'm not saying this is right, but it's how it's done. This was part b) of the question but many would have been thrown trying to answer part a) and not got that far.
4. Show what?
Show what? Another problem lies with the word ‘show’. What
does that mean in maths? Draw a diagram? Solve the equation? Come to a
conclusion? Provide a proof? It’s a vague term that should have been made much clearer. I know this is common in GCSE papers but more exact instructions would help. For a good discussion of the 'show' issue in maths see this TES piece.
5. Position
The question was not at at the end of the paper but earlier than expected for such a difficult test item (19/25). Students are taught that the questions ramp up in difficulty, with questions like these right at the end. By appearing relatively early in the paper it would have stumped many and lost them time and confidence for the completion of the last six questions. This, for me, is the one reason the grade boundaries should be reconsidered.
5. Position
The question was not at at the end of the paper but earlier than expected for such a difficult test item (19/25). Students are taught that the questions ramp up in difficulty, with questions like these right at the end. By appearing relatively early in the paper it would have stumped many and lost them time and confidence for the completion of the last six questions. This, for me, is the one reason the grade boundaries should be reconsidered.
6. Irrelevant
I’d set a high probability on the fact that the majority of politicians, supporting the maths obsession, and people working in the Department of Education, wouldn’t be able to and don’t have to be able to complete this problem. I’d say with huge certainty that the vast majority of adults, functioning well in their lives and jobs, would not be able and don’t have to be able to complete the problem. The vast majority of adults will never have to do algebra anywhere near this level of sophistication in their lives. I’d go even further and say with a very high probability that no human being has ever thought of this particular exercise as a problem, never mind used it in any practical sense. Let’s suppose we put relevance issues to one side, it is still a poorly designed test item.
7. Non-functional
Even those who do go on to higher education do not need to be able to solve this problem. In fact, the question may have skewed the Edexel exam away from the other boards Maths exams, unfairly hitting those who just happened to find that their school went with this exam board. This is a serious weakness in the English education system. We have been driven towards abandoning vocational skills, which largely need ‘functional’ maths, not the obscure algebra embedded in the GCSE curriculum. We are now locking students into a ‘resit until you pass’ model that does nothing more than make them feel like failures and turns them off progression in their education. The sooner we have a functional (not foundation) maths qualification, alongside the GCSE the better.
Maths in schools
The issue should be made clearer when the stats emerge for student performance on this question and the paper as a whole. I’ve taught maths like this to teenagers who don’t see the
point of it all, and they have a point. First, as I have argued, this question
raises the question of the quality of our exam boards and examiners. We have had impossible to answer questions and although this is just a
badly constructed test item, quality control is clearly an issue. More importantly it is a symptom of something
far worse – our modern obsession with maths in schools.
Quality
We really do have to ask whether we need multiple examination bodies in England. It increases the probability of mistakes being made and make no mistake, they are being made. This is not a one-off incident. AQA this year posed a chemistry question, then gave the full answer just a few questions later. We have had OCR ask and impossible to ask Maths question in an AS paper. The question as printed asked candidates to verify the shortest route, for two given conditions, giving values of 32.4 + 2x km and 34.2 + x km. These values should have been 34.3 + 2x km and 36.1 + x km respectively. The error was not to have included twice the journey between A and B (0.9 km) and the journey between F and G (1.0 km) in the values given. More errors were found found in A-level physics and a GCSE Latin paper from the OCR exam board and an AQA maths GCSE foundation paper. AQA admitted they had another rogue question in this year's A-level paper. Ofqual have proven to be toothless, all too ready to defend the hapless examiners and examination boards, when students were clearly affected by these errors. The quality control is clearly insufficient, realt tests by real students is nor rigorous enough and poof-checking lax.
Quality
We really do have to ask whether we need multiple examination bodies in England. It increases the probability of mistakes being made and make no mistake, they are being made. This is not a one-off incident. AQA this year posed a chemistry question, then gave the full answer just a few questions later. We have had OCR ask and impossible to ask Maths question in an AS paper. The question as printed asked candidates to verify the shortest route, for two given conditions, giving values of 32.4 + 2x km and 34.2 + x km. These values should have been 34.3 + 2x km and 36.1 + x km respectively. The error was not to have included twice the journey between A and B (0.9 km) and the journey between F and G (1.0 km) in the values given. More errors were found found in A-level physics and a GCSE Latin paper from the OCR exam board and an AQA maths GCSE foundation paper. AQA admitted they had another rogue question in this year's A-level paper. Ofqual have proven to be toothless, all too ready to defend the hapless examiners and examination boards, when students were clearly affected by these errors. The quality control is clearly insufficient, realt tests by real students is nor rigorous enough and poof-checking lax.
Solution
1. Express two probabilities:
Hannah
takes the first sweet, so there is a 6/n chance it will be orange.
(6 oranges
and n sweets)
Hannah
takes a second sweet, so there is a 5/(n-1) chance it will be orange.
As there
are only 5 orange sweets left out of a total of n - 1 sweets.
2. Calculate two probabilities as one:
The chance
of getting two orange sweets in a row is the first probability multiplied by
the second.
Which is
6/n x 5/n–1
3. Express as a single equation:
We’re told that
the chance of Hannah getting two orange sweets is 1/3.
So: 6/n x
5/n–1 = 1/3
4. Rearrange equation:
(6x5)/n(n-1) = 30/(n2 – n) = 1/3
Or 90/(n2 –
n) = 1
So (n2 – n)
= 90
Hence: n2 –
n – 90 = 0
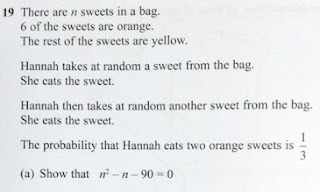

9 comments:
Besides, what does it matter that Hannah ate them?
b
> Besides, what does it matter that Hannah ate them?
Eating the sweet is a standard cue for "draw without replacement" probability problems in school maths.
Back to the main issue...
The problem is one which (better) GCSE candidates should be able to do. It just requires the student to connect three concepts which they already know: probability, formulating a mathematical statement from a word problem, and algebraic manipulation.
Unfortunately due to "teaching to the test" many will not be able do this. The ability to apply known concepts in an unfamiliar situation is fundamental to problem solving (both in life and in mathematics). If 16 year old maths students find this too difficult then where are our future mathematicians, engineers and scientists going to come from?
Let's bring some maths to that non sequitur. The fact that many found it difficult does not mean that we'll be short of mathmaticians etc. The expectation that ALL 16 year olds should know how to solve this problem is quite simply wrong.
This is an A*/A grade question definitely. But I don't think mixed-concept questions should be absent from a GCSE. I would really worry if better students couldn't answer this, especially as you are given the final answer as a 'show that...'.
Also, the quadratic does have to be solved, but only in part b which you don't reproduce. I was taught to factorise the numerical figure as a starting point, and once you go 90=10x9 (which you would do first), the answer can only be one thing.
And finally, timing questions, leaving questions and coming back to them and so on is part of exam technique.
Agree with mixed questions but the fact that so many students complained was interesting. I was trying to unpack that issue, as in my experience this doesn't suggest stupid students but faulty test items. I know that the quadratic is mentioned in b) but one possible point is that the presentation of the question threw them, along with its position in the 25 question sequence. Data from that question and paper in general will be interesting.
Having used lots of past papers I do get a sense that the examiners are detached and don;t understand context (hence the 'sweets' questions). They are also weak in terms of relevance and the structure of test items, as well as instructions. Remember that these exam boards do set questions that are literally impossible to answer - their quality control is awful.
I do not agree with your reply to my earlier post (post 2). I really find it quite offensive, and it is based on a misreading of what I wrote.
> Let's bring some maths to that non sequitur. The fact that many found it
> difficult does not mean that we'll be short of mathmaticians etc. The
> expectation that ALL 16 year olds should know how to solve this problem
> is quite simply wrong.
I did not say that "ALL 16 year olds should know how to solve this problem",
I said that the better ones should be able to do so. For one thing I believe there are two tiers of maths GCSE and this question was on the higher tier.
With regards to the twitter storm, I would suggest that the placing of the question three quarters of the way through the paper means that anyone who got this far, and answered most of the previous questions correctly, would have already achieved grades B or C. Weaker students are obviously going to find the latter part of the exam more difficult, but I don't think they would all take to the internet to complain. I therefore feel that complaints are mainly made by those who were on target for an A/A*, but were thrown by a question not following the format of previous exams.
I know you shouldn't read too much into individual comments, but can I draw your attention to these two from the Guardian.
> Sorry, you lost me at 6/n x 5/n–1.
> Its moments like this when I wonder how I got an A in GCSE Maths.
That is quite disturbing.
> Right. I was in that exam, and that part of the question was no more than
> 3 marks. It wasn't a hard question, and it wasn't worth many marks, so this
> outrage that seems to have occured is just ridiculous. Surely we should be
> talking about the questions which were worth 5 marks and were indredibly
> difficult. Take, say, the question about a seed hopper. That was difficult
> and took a lot of time to complete. It was worth a lot of marks.
Perhaps I shouldn't have mentioned the future mathematical needs of the UK. I think I must have been channelling my inner Gove. Nevertheless, with regards to future supply of STEM undergraduates I refer you to ACME report "Mathematical Needs - Mathematics in the workplace and in Higher Education" from June 2011, which states (page 15)
[continued in next post due to word count limitation]
"If 16 year old maths students find this too difficult then where are our future mathematicians, engineers and scientists going to come from?" Sorry Jihn - didn't mean to be offensive." I think it's fine and natural that the majority of 16 year olds find this difficult. I just dont agree tat the GCSE, and algebra, is the solution to the 'maths in the workplace' problem. I've been involved with this issue for many years. We have low numeracy in the workplace, on that we can agree, but this has little to do with algebra. The problem we have is not more students into HE but a decimated vocational system, where functional maths is the the real need.
The examination question about Hanna's sweets is nonsense. The probability of any of the sweets being orange is 6/n. That applies to all of them, including the first one taken out and the second and any subsequent ones. The probability of the first and second one both being orange is 36/(n x n) and that can never be 1/3 since n is an integer.
When the first sweet is removed you don't have to recalculate the probability of those remaining being orange, that probability remains unchanged at 6/n for each of them.
If you do want to recalculate after the first one is removed, then the number remaining is n-1, and the number of orange sweets remaining is (6-6/n) and so the probability of the second one being orange is(6-6/n)/(n-1) which simplifies to 6/n.
The quadratic equation is also nonsense since it is directly related to the false 1/3.
Post a Comment